lobiltd.blogg.se

An impor­tant part of ini­tial math­e­mat­ics edu­ca­tion is inte­grat­ing the abil­i­ty to count with the notion of com­par­i­son. The com­par­i­son between quan­ti­ties is present before the chil­dren start school (“why did they give you more cook­ies than me?”). This means deter­min­ing if two quan­ti­ties are equal or if one is greater than the oth­er. An impor­tant part of quan­ti­ta­tive descrip­tions is the abil­i­ty to com­pare quan­ti­ties. Humans devel­oped num­bers pri­mar­i­ly to describe the size of a set of objects and the results of mea­sure­ments. Download the PDF file here Download “Comparing Quantities” Comparing quantities in the educational context Chil­dren under­stand what it means to order num­bers with greater than, less than, or equal to is almost more impor­tant than that they know how to rep­re­sent it correctly. It is good to work with cards that include these sym­bols, so they can eas­i­ly mem­o­rize them. You likely need more context to how large x needs to be for a particular application.Sym­bol­ic rep­re­sen­ta­tion of equal, greater, or less can be com­pli­cat­ed for chil­dren. The sine approximation is even better, with error on the order of x³.īut if an approximation holds for x ≫ 1, there’s often an implicit asymptotic series in the background, and these are more subtle. That’s the case in our square root approximation above. If an approximation holds for | x| ≪ 1, there’s often an implicit power series in the background, and the error is on the order of x². It’s often harder to tell from context when something is large than when it is small. The error in the example is more than 30,000, but this value is small relative to 10! = 3,628,800. In Math, while comparing the numbers, we will use mainly three symbols: greater than, less than and equal. We will compare the numbers such as natural numbers, whole numbers, integers, fractions, and decimal numbers in mathematics. The relative error is small, not the absolute error. Greater than \(( > )\) Less than \(( < )\) Equals to \(( )\) Practice Exam Questions. Note that the approximation error above is small relative to the exact value. For instance, if n = 10, the approximation above has an error of less than 1%.

Sometimes you see something like n ≫ 1 to indicate that n must be large. How small is small enough? The post explains how to know. A lot of people memorize “You can replace sin θ with θ for small angles” without thoroughly understanding what this means. If θ is small, the error in the approximation above is very small.Ī few years I wrote a 700-word blog post unpacking in detail what the previous sentence means. Rather than saying a variable is “small,” we might say it is much less than 1. The ratio b/a = 0.03, and your error should be small relative to 0.03, so the approximation above should be good enough. Suppose you need to know √103 to a couple decimal places. If, in your context, you decide that b/ a is small, the approximation error will be an order of magnitude smaller. So when is | b| much less than a? That’s up to you.

You might see somewhere that for | b| ≪ a, the following approximation holds:

All jargon is like this.īelow are some examples of ≪ and ≫ in practice. You have to know the context to understand how to interpret them, but they’re very handy if you are an insider. The symbols ≪ and ≫ can make people uncomfortable because they’re insider jargon. Sometimes you’ll see ≫, or more likely > (two greater than symbols), as slang for “is much better than.” For example, someone might say “prototype > powerpoint” to convey that a working prototype is much better than a PowerPoint pitch deck. Is 5 much less than 7? It is if you’re describing the height of people in feet, but maybe not in the context of prices of hamburgers in dollars. Here’s a little table showing how to produce the symbols.

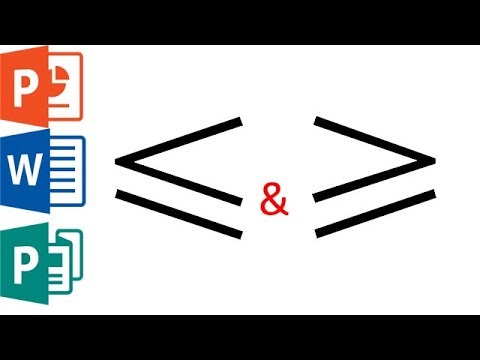
The symbol ≪ means “much less than, and its counterpart ≫ means “much greater than”. The symbols ≪ and ≫ may be confusing the first time you see them, but they’re very handy.